What is Dyscalculia?

DYSCALCULIA is a specific learning difficulty with mathematics, primarily arithmetic. It was defined in a UK Government document in 2001 as: 'Dyscalculia is a condition that affects the ability to acquire mathematical skills. Dyscalculic learners may have a difficulty understanding simple number concepts, lack an intuitive grasp of numbers, and have problems learning number facts and procedures Even if they produce a correct answer or use a correct method, they may do so mechanically and without confidence.'

It is defined in the USA (Diagnostic and Statistical Manual of Mental Disorders, 2013) as:
'Difficulties in production or comprehension of quantities, numerical symbols, or basic arithmetic operations that are not consistent with the person's chronological age, educational opportunities, or intellectual abilities.'

Research into dyscalculia has lagged far behind research into dyslexia, but this is beginning to even out. Researchers agree that there is no single profile of dyscalculia. It is a heterogeneous problem which is compounded by the constellation of demands made by maths with prevalence at around 5%.

Key Indicators of Difficulty

An over-reliance on counting in ones.

Use manipulatives, such as base ten blocks to provide visual images of quantities. Use recognisable and consistent patterns based on 1, 2, 5 and 10.

For example;

Children can be shown how 'chunking' can help them work beyond ones.

Difficulty in counting backwards.

Counting backwards requires working memory, so it can be much harder than many teachers and parents recognise. It sets the foundation for subtraction. Use materials and visuals again. It's a good opportunity to introduce and demonstrate vocabulary such as 'take away' and 'subtract' and one of my favourite questions, 'Is it bigger or smaller?'

A poor sense of number and an inability to estimate.

Again objects set out in recognisable patterns create the foundations for developing this skill. Try comparing objects set out in patterns with random sets. That question, 'Is it bigger or smaller?' is useful again.

Difficulty in understanding place value and its role in arithmetic.

A child can learn to count to ten, but writing ten as 10 is a very sophisticated task in terms of understanding how that communicates 'ten'. This can be demonstrated as a cognitively developmental sequence, using base ten blocks on a place value card as the starting point and then weaning the child down to using the symbols (digits) on their own.

The processes of multiplying and dividing by 10, 100, 1000 and so on can be demonstrated with a similar process.

Poor recall of basic facts, but better with 2x, 5x and 10x facts.

All facts are useful, but some are more useful than others. Luckily the more useful ones are the ones learners are most likely to learn. Children can be shown how to link the patterns (and their symbols) to demonstrate addition and subtraction facts, for example,

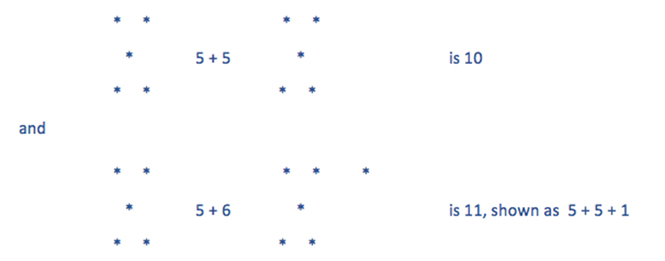
The principle can be extended to multiplication facts. Understanding is developed as well as strategies for accessing facts. Multiplication is linked to addition, so 7 x 6 is explained and demonstrated as repeated addition of six sixes, 6 + 6 + 6 + 6 + 6 + 6 + 6. The sixes can be chunked as (6 + 6 + 6 + 6 + 6) and (6 + 6), that is 5 x 6 and 2 x 6, making 30 + 12, making 42.

This strategy can be applied to many other examples and it teaches the child about multiplication and how it is linked to addition. It sets the foundation for later, more challenging work on multiplication.

Slow speed of working.

There are a number of contributing factors that combine to make dyscalculic children slower to do maths problems, for example, slow retrieval of basic facts and slow and uncertain recall of procedures. So they do fewer examples and gain less experience. They become more anxious and that makes the situation worse. So they need less examples, but carefully selected to provide a sufficient breadth of learning experience.

The culture of maths to provide answers quickly is counter-productive for many children. Any help in reducing this (often unrealistic) expectation will be good for the child.

Very weak skills for mental arithmetic.

There are people who think that mental arithmetic skills are an essential basic for learning maths. I would challenge that belief. Two skills you need to be good at mental arithmetic are short term memory (to remember the question) and working memory (to work out the answer). Dyscalculic children often have weak short term and working memories. A reasonable adjustment would be to show the question, not just say the question. Another reasonable adjustment would be to give more time and that means more than an extra 25%.

Task avoidance.

If a child (or adult) predicts that they will get a task wrong, then they will, quite reasonably, avoid it. This is a fear of negative evaluation. Most of us experience this about something. If you don't try you don't fail. That requires setting a classroom, and home, ethos that allows a child to fail without that failure creating another step up the withdrawal ladder. High levels of anxiety, usually specific to maths.

Anxiety can be facilitative. That rarely happens for dyscalculic students. There is sound research that has demonstrated that anxiety makes working memory less effective and thus reduces the ability to do maths. In my school we used attributional style to help tackle fear of failure, anxiety and low self-esteem.

Forgets maths procedures and formulas.

If long term memory for maths is poor, then the child needs support to remember the often quite complex formulas we use in maths. It is a huge help if the child understands what he is doing and why. The steps should make sense and the task as a whole should make sense. Since maths builds, this will usually require a sound understanding of the pre-requisite work. Hence the need to revise, again and again. It is of no use what-so-ever to say to a child, 'I've told you this ten times, you should remember it.' Ten times without understanding is a waste of times. Use materials and visuals (alongside the symbols and digits) to develop understanding.

It is, as Margaret Rawson said, a matter of teaching maths as it is to the child as he is.' You need to understand both.

More details on methods for intervention can be found in the BDA book, 'Maths Learning Difficulties, Dyslexia and Dyscalculia' and Steve's video tutorials, 'Maths Explained', both available via the BDA.

Top ten Tips for teaching children with dyscalculia

1. Use concrete materials, such as Cuisenaire rods or base ten materials
2. Spend time exploring these and don't take them away too soon, they will help to develop the child's understanding.
3. Play games with dice and dominoes so that the child can recognise common dot patterns.
4. Try to encourage the child to use more efficient calculating strategies, such as counting on rather than counting all.
5. Encourage the child to visualise the maths- by drawing diagrams and using concrete materials to model the maths.
6. Make the maths practical and multisensory- avoid worksheets.
7. Spend time on place value so that it is fully understood, this can be a very difficult concept to grasp.
8. Have a little and often approach- repetition and 'overlearning' will help.
9. Use mathematical language as much as possible and encourage the child to do the same.
10. Give multiplication grids and number bonds to reduce the stress of having to remember these facts.